3.8 Stoichiometry

Stoichiometry is the accounting of atoms before and after a chemical reaction. It is an expression of the Law of Mass Conservation, in that elements are neither created nor destroyed in a chemical reaction, and that mass is an intrinsic property of every element. Thus, the numbers, types of atoms, and total mass exiting a chemical reaction (i.e. the “products” of that reaction) must be the same as the numbers, types of atoms, and total mass entering that chemical reaction (i.e. the “reactants”). For example, in the combustion of natural gas in an oxygen-rich environment, the fuel (CH₄) and oxidizer (O₂) are the reactants, while water vapor (H₂O) and carbon dioxide gas (CO₂) are the products:

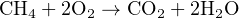
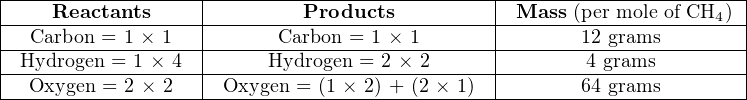
As you can see in this example, every single reactant atom (and its mass) entering the reaction is accounted for in the product molecules. The only exception to this rule is in nuclear reactions where elements transmutate into different elements, with gains or losses in nuclear particles. No such transmutation occurs in any mere chemical reaction, and so we may safely assume equal numbers and types of atoms before and after any chemical reaction. Chemical reactions strictly involve re-organization of molecular bonds, with electrons as the constituent particles comprising those bonds. Nuclear reactions involve the re-organization of atomic nuclei (protons, neutrons, etc.), with far greater energy levels associated.

Often in chemistry, we know both the reactant and product molecules, but we need to determine their relative numbers before and after a reaction. The task of writing a general chemical equation and then assigning multiplier values for each of the molecules is called balancing the equation.

3.8.1 Balancing chemical equations by trial-and-error

Balancing a chemical equation is a task that may be done by trial-and-error. For example, let us consider the case of complete combustion for the hydrocarbon fuel ethane (C₂H₆) with oxygen (O₂). If combustion is complete, the only products will be water vapor (H₂O) and carbon dioxide (CO₂). The unbalanced equation representing all reactants and products for this reaction is shown here, along with a table showing the numbers of atoms on each side of the equation:

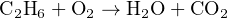
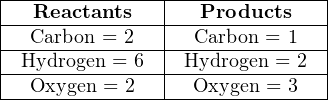
Clearly, this is not a balanced equation, since the numbers of atoms for each element are unequal between the two sides of the equation.

A good place to start in balancing this equation is to look for an element represented by only one molecule on each side of the equation. Carbon is an example (present in the ethane but not in the oxygen molecule on the left-hand side, and in the carbon dioxide but not the water on the right-hand side) and hydrogen is another.

Beginning with carbon, we see that each ethane molecule contains two carbon atoms while each carbon dioxide molecule contains just one carbon atom. Therefore, we may conclude that the ratio of carbon dioxide to ethane must be 2-to-1, no matter what the other ratios might be. So, we double the number of carbon dioxide molecules on the right-hand side and re-check our atomic quantities:

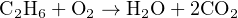
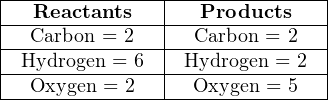
Next, we will balance the hydrogen atom numbers, since we know hydrogen is an element found in only one molecule on each side of the equation. Our hydrogen ratio is now 6:2 (left:right), so we know we need three times as many hydrogen-containing molecules on the right-hand side. Tripling the number of water molecules gives us:

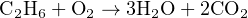
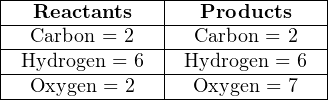
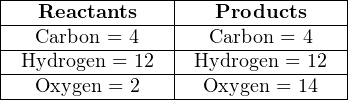
Unfortunately, the numbers of oxygen atoms on each side of the equation are unequal, and it is not immediately obvious how to make them equal. We need five more atoms of oxygen on the left-hand side, but we cannot add exactly five more because oxygen atoms only come to us in pairs (O₂), limiting us to even-number increments.

However, if we double all the other molecular quantities, it will make the disparity of oxygen atoms an even number instead of an odd number:

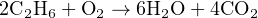
Now it is a simple matter to balance the number of oxygen atoms, by adding six more oxygen molecules to the left-hand side of the equation:

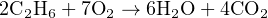
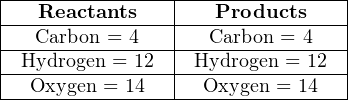
Now the equation is balanced: the quantities of each type of atom on both sides of the equation are equal.

3.8.2 Balancing chemical equations using algebra

A more mathematically sophisticated approach to stoichiometry involves the use of simultaneous systems of linear equations. The fundamental problem chemists must solve when balancing reaction equations is to determine the ratios of reactant and product molecules. If we assign a variable to each molecular quantity, we may then write a mathematical equation for each element represented by the reaction, and use algebra to solve for the variable values.

To illustrate, let us balance the equation describing the attack of aluminum metal’s protective “passivation” layer of oxide by acid rain. When aluminum metal is exposed to oxygen, the outer surface of the metal quickly forms a layer of aluminum oxide (Al₂O₃) which acts to impede further oxidation of the metal. This protective layer, however, may be attacked by the presence of sulfuric acid (H₂SO₄). This acid finds its way into rainwater by way of sulfur compounds emitted during the combustion of sulfur-laden fuels. The products of this reaction between sulfuric acid and aluminum oxide are a sulfate molecule (Al(SO₄)₃) and water (H₂O), as illustrated in this unbalanced chemical equation:

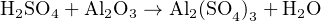
This equation contains four different compounds (acid, aluminum oxide, sulfate, and water), which means we ultimately must solve for four different multiplier quantities. It also contains four different elements (H, S, O, and Al). Since the mathematical requirement for solving a system of linear equations is to have at least one equation per variable, it would first appear as though we could set up a 4 × 4 matrix (four equations of four variables). However, this will not work. If we tried to solve for four unknown quantities, we would ultimately be foiled by an infinite number of solutions. This makes sense upon further inspection, since any stoichiometric solution to this chemical reaction will have an infinite number of correct proportions to satisfy it²⁷ . What we need to do is arbitrarily set one of these molecular quantities to a constant value (such as 1), then solve for the quantities of the other three. The result will be ratios or proportions of all the other molecules to the fixed number we assigned to the one molecule type.

As an example, I will choose to set the number of acid molecules to 1, and use the variables x, y, and z to solve for the numbers of the other molecules (oxide, sulfate, and water, respectively):

Now, I will write four algebraic equations, each algebraic equation representing the stoichiometric balance of a single element in the chemical equation. Focusing on the element hydrogen as an example, there will be two hydrogen atoms for every one molecule of acid, 0x hydrogen atoms for every x molecules of aluminum oxide, 0y atoms of hydrogen for every y molecules of aluminum sulfate, and 2z atoms of hydrogen for every z molecules of water. The following table shows each of the four elements with their respective balance equations:

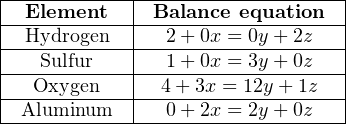
Simplifying each equation by eliminating all zero values and “1” coefficients:

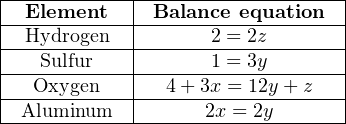
We can see by examination of the first, second, and fourth equations that z must be equal to 1, y must be equal to 1 3, and that x and y are equal to each other (therefore, x must be equal to 1 3 as well). Plugging these values into the variables of the third equation confirms this (4 + 1 = 4 + 1). Thus, our solution to this multi-variable system of equations is:

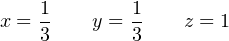
It makes little sense to speak of fractions of a molecule, which is what the values of x and y seem to suggest, but we must recall these values represent proportions only. In other words, we need only one-third as many oxide and sulfate molecules as acid and water molecules to balance this equation. If we multiply all these values by three (as well as the initial constant we chose for the number of acid molecules), the quantities will be whole numbers and the chemical reaction will still be balanced:

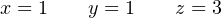
Thus, our final (balanced) equation showing the attack of aluminum metal’s passivation layer by acid rain is as follows:

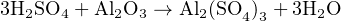
Another example to illustrate this method of balancing chemical equations is the oxidation of wastewater (sewage) sludge. Here, the reactant is not a single type of molecule, but rather a complex mixture of carbohydrates, proteins, fats, and other organic compounds. A practical way of dealing with this problem is to represent the average quantities of carbon, hydrogen, and oxygen in the form of a compositional formula²⁸ based on a gross analysis of the wastewater sludge:

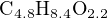
We know that the products will be carbon dioxide and water, but the question is how much oxygen will be required to completely oxidize the mixture. The following (unbalanced) chemical equation shows the reactants and products:

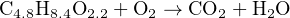
The non-integer subscripts greatly complicate trial-and-error stoichiometry, but they pose absolutely no obstacle at all to simultaneous equations. Assigning variables x, y, and z to the unknown molecular quantities:

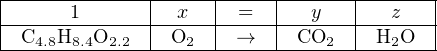
Now, we may write three algebraic equations, each representing the stoichiometric balance of one element in the chemical equation. The following table shows each of the three elements with their respective balance equations:

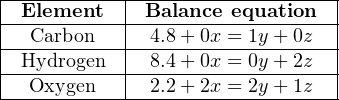
Simplifying each equation by eliminating all zero values and “1” coefficients:

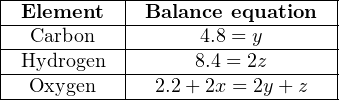
We may tell from the first and second equations that y = 4.8 and z = 4.2, which then leads to a solution of x = 5.8 once the values for y and z have been inserted into the third equation. The final result is this balanced compositional equation for the oxidation of wastewater sludge:

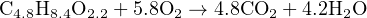
My own personal experience with the use of simultaneous linear equations as a tool for stoichiometry is that it is much faster (especially when balancing complex reaction equations) than trial-and-error, and relatively easy to set up once the general principles are understood.

3.8.3 Stoichiometric ratios

Regardless of the technique used to balance the equation for a chemical reaction, the most practical purpose of balancing the equation is to be able to relate the reactant and product quantities to each other. For instance, we may wish to know how much oxygen will be required to completely combust with a given quantity of fuel, so that we will be able to engineer a burner system capable of handling the necessary flow rates of fuel and oxygen. Balancing the chemical reaction is just the first part of the solution. Once we have a balanced equation, we need to consider the ratios of the substances to each other.

For example, let us consider the balanced (stoichiometric) chemical equation for the combustion of ethane fuel with pure oxygen:

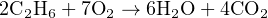
From the balanced chemical equation we can see that for every 2 molecules of ethane, we will need 7 molecules of oxygen gas to completely combust, producing 6 molecules of water vapor and 4 molecules of carbon dioxide gas. The numerical multipliers preceding each molecule in the balanced equation tell us the molar ratios of those substances to each other. For oxygen to ethane the ratio is 7:2, for water to ethane the ratio is 6:2 (or 3:1), for carbon dioxide to water the ratio is 4:6 (2:3), etc. If for some reason we needed to calculate the number of moles of CO₂ produced after burning 80 moles of ethane, we could easily calculate that by multiplying the 80 moles of ethane by the 2:4 (1:2) ethane-to-carbon dioxide ratio to arrive at a figure of 160 moles of CO₂. If we wished, we could even solve this using the same method of unity fractions we commonly apply in unit-conversion problems, writing the carbon dioxide-to-ethane ratio as a fraction of two equivalent quantities:

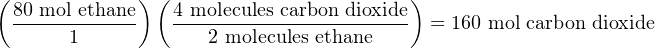
If any substances involved in the reaction happen to be gases at nearly the same pressure and temperature²⁹ , the molar ratios defined by the balanced equation will similarly define the volumetric ratios for those substances. For example, knowing our ideal oxygen-to-ethane molar ratio is 7:2 tells us that the volumetric flow rate of oxygen to ethane should also be (approximately) 7:2, assuming both the oxygen and ethane are gases flowing through their respective pipes at the same pressure and at the same temperature. Recall that the Ideal Gas Law (PV = nRT) is approximately true for any gas far from its critical phase-change values. So long as pressure (P) and temperature (T) are the same for both gases, each gas’s volume (V ) will be directly proportional to its molar quantity (n), since R is a constant. This means any molar ratio (n₁ n₂ ) for two gases under identical pressure and temperature conditions will be equal to the corresponding volumetric ratio (V ₁ V ₂ ) for those gases.

It is important to understand that these molar ratios are not the same as the mass ratios for the reactants and products, simply because the different substances do not all have the same mass per mole.

If we regard each of the multipliers in the balanced equation as a precise molar quantity (i.e. exactly 2 moles of ethane, 7 moles of oxygen, etc.) and calculate the mass of the reactants, we will find this value precisely equal to the total mass of the products because the Law of Mass Conservation holds true for this (and all other) chemical reactions:

Calculating mass based on 2 moles of ethane, we have a total reactant mass of 284 grams (60 grams ethane plus 224 grams oxygen), and a total product mass of 284 grams as well (108 grams water plus 176 grams carbon dioxide gas). We may write the mass ratios for this chemical reaction as such:

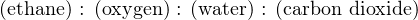
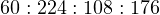
If for some reason we needed to calculate the mass of one of these substances in relation to the other for this reaction, we could easily do so using the appropriate mass ratios. For example, assume we were installing a pair of mass flowmeters to measure the mass flow rates of ethane and pure oxygen gas flowing into the combustion chamber of some industrial process. Supposing the ethane flowmeter had a calibrated range of 0 to 20 kg/min, what range should the oxygen’s mass flowmeter be calibrated to in order to match in perfect stoichiometric ratio (so that when one flowmeter is at the top of its range, the other flowmeter should be also)?

The answer to this question is easy to calculate, knowing the required mass ratio of oxygen to ethane for this chemical reaction:

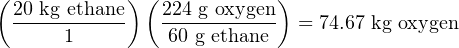
Therefore, the oxygen mass flowmeter should have a calibrated range of 0 to 74.67 kg/min. Note how the unit of mass used in the initial quantity (20 kilograms ethane) does not have to match the mass units used in our unity fraction (grams). We could have just as easily calculated the number of pounds per minute of oxygen given pounds per minute of ethane, since the mass ratio (like all ratios) is a unitless quantity³⁰ .

Back to Main Index of Book